Cuisenaire Rods – The best manipulative of them all?!?

Today I received a really exciting package. I bought my own set of Cuisenaire Rods! For those that have not heard of these they are sometimes called Proportional Rods as they are used to illustrate proportional reasoning, amongst other concepts.

I remember these from my first day at school. We were given these rods and told to play with them. It is a really clear memory and I have loved these rods ever since.

Unfortunately, they have fallen out of fashion since then (it was a very long time ago) and now there are countless boxes at the back of school cupboards gathering dust.

Have a look in your school and see if you can find some. If you can, get them out, dust them off and let’s explore some of the ways they can be used.

The basic concept is that the lengths of the rods are proportional to each other. Note the colours are important too. Although the originals are wooden you can now get them in plastic and virtually too. No matter what material yours are they should all have the following properties:

White – 1cm in length (a cube)
Red – 2cm in length
Light Green – 3cm
Purple – 4cm
Yellow – 5cm
Dark Green – 6cm
Black – 7cm
Brown – 8cm
Blue – 9cm
Orange – 10cm

The connections between the colours is as follows:

White (1) and Black (7) are on their own
Red (2), Purple (4), Brown (8) – Red family – multiples of 2
Light Green (3), Dark Green (6), Blue (9) – Blue family – multiples of 3
Yellow (5), Orange (10) – Yellow family – multiples of 5

When students are first introduced to these rods, they are told the values as 1, 2, 3 . . . But it is important to note that this is NOT the only way they can be seen. By being flexible with the values many more concepts can be explored.

I am going to demonstrate a (very) few of the ways in which Cuisenaire rods can be used below and post links to websites where you can continue exploring. These are NOT tools for the very young grades only. I believe that they should be used in older grades too. Especially when exploring fractions and decimals, as I will demonstrate below.

Ideas for the younger grades:

By laying down a rod and then finding combinations of other rods that make the same length students can explore addition facts, for any number.

Here are some arrangements for Facts to 5

Students can be asked questions such as: Are these two arrangements the same?

They both make 5 and use the same smaller rods but are they the same? This can help students to understand the communicative and associative properties of addition.

Use the rods to explore odd and even numbers and what happens when you add odds and evens.

They can be used to model subtraction problems: Here is 7 – 4

There are several ways to discover the difference:

Multiplication as repeated addition is easily modelled too.

Ask the question – Can we use only one other rod to reach the same length?

What does this tell us?

Other ideas for younger grades:

Partitioning

Make a picture worth 100 or 50 . . .

Barrier games – students describe what pattern/picture they have made and another has to form it from the verbal instructions

Finding areas – great for introducing the area model for multiplication

Factorising

A picture worth 100

For the older grades

When working with older grades we can play around with the values of the rods.

Call the Orange rod ONE – now what are the values of the other rods?

Call the Yellow rod ONE – now what are the values of the other rods?

This is a great way for students to explore fractions and decimals.

If the Purple rod has the value ½ or 0.5, what are the values of the Red rod and the White rod? What is the value of the Blue rod?

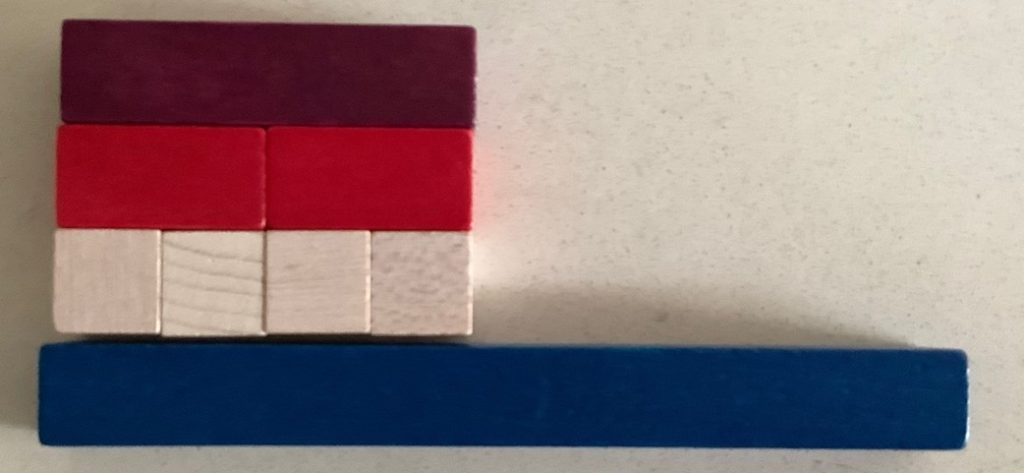
There are so many other ways to use these rods, I am sure that students themselves, once they are familiar with them and comfortable with using them, will find uses you had not thought of.

The most important thing is to give the rods to students, allow them to ‘play’ with them for a while. Then use them regularly for specific activities. If they are left out and are easily available students will use them when they feel the need. They are not just for ‘weaker’ students but there as a tool to support all students!

Resources

History:

https://en.m.wikipedia.org/wiki/Cuisenaire_rods

https://youtu.be/ae0McT5WYa8

Nrich:

https://nrich.maths.org/search/?search=Cuisenaire&tab=1&fs=111110000000111

Paul Swan:

https://drpaulswan.com.au/shop/reasoning-with-rods/

NZMaths:

https://www.tki.org.nz/tki-content/search?SearchText=Cuisenaire&SearchDate=%5B%5D&SearchButton.x=14&SearchButton.y=19&TKIGlobalSearch=1

Calculate:

https://calculate.org.au/?s=cuisenaire&security=9a13025314&_wp_http_referer=%2F

Virtual Cuisenaire Rods:

https://mathsbot.com/manipulatives/rods

Number Talks – Why Maths Should be Noisy

Why we need to do Number Talks

One of the proficiencies in the Australian Curriculum is reasoning. This is a tricky aspect of mathematics to assess and to ‘see’ happening in a student’s mind.

Too often our students seem to think that in maths they do not need to write sentences, or even to think in sentences.

I believe that this is partly due to the worksheet culture that has seeped into maths teaching. Even in the virtual and online maths programs that are now being promoted the aim is to type in the correct number, no other information is needed, to win or to move on to the next question. Maths textbooks, for the primary years, are often just a collection of worksheets with boxes for the students to fill in.

Even NAPLAN encourages this perception. All the student must do is to write/type in the number. They are not even required to give the correct unit (if needed) as this is already there.

When students move into high school and are confronted by a textbook that is not also a workbook and have to start setting out their work and writing down their thinking, this is extremely challenging for many.

We, teachers, need to create a safe space where students can express and share their thinking. Where they can explore ideas and see alternative ways of approaching problems.

This is where I believe Number Talks come in to play.

Number Talks – what are they?

For those that are not familiar with these here is a (very) quick description:

A problem or image is shown to the students
Students have time to look at it and to come to an answer
The teacher then notes down the students’ answers
The students are then invited to describe their thinking – how they got their answer

Some important points to note:

The teacher should not pass judgement when collecting the students’ responses to the problem. This is difficult. Teachers are conditioned to react. Either by praising when a student is correct or asking for another response when a student is wrong. Keeping a ‘poker face’, not showing any reaction, as the students give their responses is not easy and needs to be practiced.
Calculations should be presented horizontally. This is important as the main aim of a number talk is to encourage different ways of thinking. If a problem is presented vertically most students will try to complete the problem using a familiar algorithm.

This is a mental activity. The students should be solving the problem in their head. (Though I do believe that a mini-whiteboard, Rekenrek or bead-string should be allowed if the problem is complex or the students ask for them.)
See the references below for more on how to run a Number Talk.

What Number Talks can bring to the classroom

Number Talks can bring a wealth of benefits to the classroom. Below are a few:

Vocabulary

Giving students the correct mathematical terminology and allowing them to use it in context is important. I think we adults underestimate kid’s ability to understand and use sophisticated terms. There is no reason why we cannot teach the terms commutative and associative when we first introduce the concepts. If the teacher is using these terms, in context, regularly the students will too.

Reasoning

As I mentioned above allowing students time to think through a problem and then to express it verbally expands their ability to reason logically.

So often when asked how they know that is the answer students will respond ‘I just know it’.

The Australian Curriculum in its description of what reasoning is uses the verbs: justify, proving, explaining. We need to teach these skills from Foundation. To use this vocabulary and to give the students a forum where they can demonstrate these skills.

Communication

I titled this blog post – why maths should be noisy. I remember my own maths classes in high school. The teacher would talk (well lecture really). A few questions would be answered (only if you put your hand up and did not call out) and then we would sit, in silence, and complete an exercise. This was the model of a good maths lesson.

I feel that the classroom should be noisy with a lot of discussion and, on-task, chatter. Mathematicians do not work in a vacuum. Very few people do.

“If you can teach it you know it” is a phrase I often say to students when they ask how to revise for a test. Similarly, if a student can convince others that their answer is correct and that their method is sound it shows that they have a deep understanding that they can communicate.

Not a Number Talk

When we say ‘Number Talk’ a certain format comes to mind. The structure, use of hand signals etc. But we should be looking at how to get our students talking, thinking, reasoning, communicating more. A ‘Notice and Wonder’ or ‘Which One Does not Belong’ or just a problem from the textbook can be displayed and used a the starting point.

A Number Talk does not just have to follow the traditional format.

For instance, Jo Boaler recently added to her Youcubed site Data Talks, extending this format in to another area of mathematics, away from just number.

Start every lesson with a problem, allow time for thinking and then for discussion and sharing of ideas.

Our lessons should be filled with talk!

References:

http://www.meaningfulmathmoments.com/number-talks.html

https://www.youcubed.org/resource/data-talks/

http://wodb.ca/

https://www.nctm.org/Classroom-Resources/Problems-of-the-Week/I-Notice-I-Wonder/

Virtual manipulatives – An exciting way to visualise maths

During the COVID-19 pandemic the educational world has under gone a huge change and shift in thinking. Many teachers have had to look for new ways to deliver concepts in maths. From the increase in activity and membership I have seen on my Facebook site there has been a renewed interest in the use of manipulatives when teaching mathematics. Using online, virtual manipulatives has given:

students a new way of thinking about and visualising maths
teachers a new way of presenting concepts

There are many students that have thrived in this new learning environment. In discussions I have had with teachers, maths seems to have been the subject where most students have made good progress while learning online. Even those students that generally struggled in the classroom. The lack of distractions the, often, smaller teaching groups and more focussed attention they have received has proved to be beneficial. I am a great believer and proponent of using concrete materials. The physical experience of touching and moving manipulatives while learning maths is an excellent way to fix ideas and concepts. During the move to online learning many teachers had to look for new ways to deliver the concepts they have been teaching for years. In their search they have discovered the myriad of virtual manipulatives that are available. Many teachers are using manipulatives more now than they did in their physical classrooms. When we return to the classroom ideally we should switch to the physical manipulative, and not just continue with the virtual. The virtual manipulative is certainly better than no manipulative, but I do not believe that it can replace the physical. The ability to make this switch will depend on the availability of concrete materials and budgetary constraints. Teachers should be looking in cupboards for those dusty Cuisenaire rods and searching for those long forgotten resources that are hiding in so many schools. Let us all use the amazing teaching and learning that we have been doing online and transfer to the classroom with the resolve to keep looking for innovative and creative ways to deliver the maths curriculum and to foster creativity and growth in our students. My situation has changed too. I am no longer working in a school and must think about what is next. I am looking to start a maths education consultancy. Taking the ideas of growth mindset in maths and the use of manipulatives to schools, students and parents. Still working on the idea. Look out for the launch in the coming weeks.

Rekenreks and Bead Strings

In the last week I have been going into classes and making manipulatives with beads. Beads are a great resource and can be used in so many ways.

Renenrek

In Foundation classes (5 year-olds) we made Rekenreks. These are like mini-abacuses with two rows of 10 beads, in two groups of five. They can be used with students who are beginning to learn to count and develop one-to-one correspondence. You can also do some great activities on partitioning.

To make these we used the following materials:

Sticky-backed, coloured foam sheets
Coloured pipe cleaners
Black and white pony beads ( Traditionally red and white beads are used, but I could not find red ones.)

We chose foam sheets and pipe cleaners in matching colours so that the beads would stand out clearly. In the example above both are green. We had a variety of colours and let the students choose which they wanted – that way those that have difficulty distinguishing colours could choose the ones that worked best for them.

The students were asked to first count out two groups of five beads in each colour, then to thread them on to the pipe cleaners – great for fine motor skills. After they were checked by a teacher the pipe cleaners were threaded into one of the foam sheets so that they had room to move. The backing was removed, the pipe cleaner stuck to the back and the second sheet then stuck to that. This made the rekenreks secure.

The students were really excited to make and then use these. We worked on showing numbers in different ways, using one or two of the rows and looking for patterns. The teachers were also excited by these and are looking forward to integrating them into their teaching.

The pipe cleaners mean that the beads do not move by themselves, the students have to push them along. This adds a kinaesthetic (tactile) dimension to the activity.

Bead Strings

In Year 1 and Year 2 (6 and 7 year-olds respectively) we made more traditional bead strings.

In Year 1 we made a string with 30 beads in two colours, in groups of 5

In the example above I used beads of a different colour at the ends to stop the beads falling off. When we did this in class I had clear beads and used these instead.

In Year 3 we made strings of 100 beads in two colours in groups of 10.

Some people make the strings into bracelets or necklaces but I like to leave them untied so they can be laid flat on a desk. Some students find them easier to use like that.

With each string we looked at:

counting in groups
which size groups left no spare beads at the end
what patterns they saw when counting
folding the strings
using the bead string as a number line

References

https://calculate.org.au/2018/12/10/bead-strings/

https://www.mathlearningcenter.org/sites/default/files/pdfs/LTM_Rekenrek.pdf

Numerule: A teacher’s review of the innovative maths tool

This year I was lucky enough to present at a few mathematics conferences. At two of them, MAWA (Mathematical Association of Western Australia) and MAV (Mathematical Association of Victoria) I presented on the topic of ‘Using Manipulatives in the High School Classroom’. I had a great response to this topic with all the presentations well attended and really good discussions.

I realised that this was a topic that teachers were interested in and I began to think of ways to continue and grow the discussion. In a moment of madness, I started the FaceBook page Maths Manipulatives and was amazed at the response. I had 50 members in 10 minutes and a request to review a product. By the end of 48 hours, I had 1400 group members and the number has been slowly growing since then.

In this post, I will be reviewing the Numerule. I first saw this at the MAV conference and thought that it looked like a clever idea.

What is a Numerule?

The Numerule is a 30cm ruler that doubles as a simple counting tool for use in Lower Primary classrooms. Corresponding to each number on the ruler is a ‘button’ that can be pressed. Once pressed the button stays down, pushing up from underneath returns it to its original state. The buttons are grouped in tens by colour (blue, white and red).

The information that comes with the Numerule describes how it can be used for the four operations as well as for recording data. There are videos on the website showing children performing these tasks. The information also includes links to many Australian Curriculum descriptors. The website also has a teacher’s notes to download.

Trying it for the first time

As I received the Numerule, after school had finished I brought it to try out with my 6-year-old granddaughter. I gave it to her to look at without telling her what it was. She immediately started to push the buttons and saw that they could be pushed back into position. She used the ruler to measure a few things and told me that it could be used to draw straight lines. I asked her about the buttons she told me that they were in groups of ten and then counted in tens to 30.

I then told her that we could use it to do some maths questions. I asked her to show me how she could do 6 plus 5. She thought for a bit and then pushed down the 6 and counted on five, pushing the buttons as she went. The answer she told me was 11. I was impressed at how intuitive she found using it. We tried a couple more questions and she enjoyed doing them.

She then said look – and pushed down every other button and showed me that this was counting in two’s. Again this was without prompting.

I then guided her a little and we looked at subtraction problems. She then took the Numerule to bed!

My impression of the Numerule

Having now seen the Numerule in action I can see that it is a good design and looks to be strong enough to last in the classroom. My granddaughter took to it and quickly saw how to use it. I can see that in the classroom this could be a useful tool. As every student should have a ruler having one that doubles as a calculation aid makes sense.

If I have any criticism it would be that for the age group it is aimed at I would have grouped the colours in fives, not tens. This is how I make bead strings with students. I like the tactile nature of pressing the buttons and the way it can be used for data collection and representation.

Also, it is a little pricey at $14 (15% less if you buy over 25) but I was told that it is virtually unbreakable so should last. My younger grandchildren had a go with it and it did stand up to some quite rough treatment.