This post will explore multiplying algebraic expressions, the area model, and factorising quadratic expressions.
Multiplying Expressions
Multiplying algebraic expressions by an integer
Here is an example of how to multiply an algebraic expression by an integer. This may seem like quite a trivial example, but students often struggle with the concept of multiplying each part of an expression. In this example, by laying out the expression and then repeating it the given number of times, they can clearly see that each part of the expression is multiplied. This will help set them up for the more complex multiplications to follow.
The Area Model
Before introducing multiplying two linear expressions, it may be useful to revise the area model with students. This model is the base from which they can visualise what is happening when they do the multiplication. Relating an algorithm to a concrete and/or visual representation will deepen the students’ understanding.
Multiplying two linear expressions
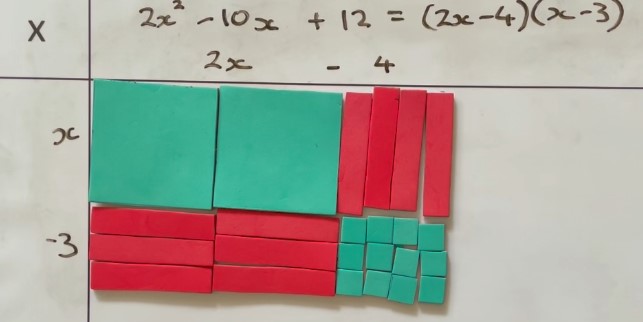
Once students have a good grasp of the area model, you can move on to multiplying two linear expressions. The example below shows how you model this by building the area indicated by the factors (the given brackets). I show how you can visually link the concrete, representational and abstract.
Allow the students to decide which method they want to use. Try not to give the impression that using the tiles means they are not as ‘advanced’ as others. Students should feel comfortable to pick up the tiles and use them if they meet a problem where they feel the tiles will help them. In other words, the tiles are a tool, just like a calculator, and students should feel as comfortable reaching for the tiles as they do reaching for the calculator. Similarly, drawing visual representations. Mini-whiteboards are great for these as they allow students to erase ‘mistakes’ and move on.
Factorising Quadratics
Factorising Linear Expressions
Introduce factorising with very simple examples. In the video, I explain that factoring is building a rectangle, with the given pieces, so that there is the same number of each piece in each layer. Doing a few, really simple, examples will help students to get the idea of what factorising is – dividing.
You may want to give students an example where there is more than one way to factorise and then you can discuss which way is ‘better’ and why.
Factorising a quadratic expression
Once students can multiply linear expressions to form quadratic expressions they can begin to explore factorising – the opposite operation.
Starting with simple quadratics that do factorise easily, as in the video below, will familiarise students with the process. I have tried to show how the area model relates to the generally taught algorithm.
In the example in the video, factorising: x2 + 5x + 6 you might explain that you are looking for:
Two numbers that multiply to +6 and that add to +5
| Possible factors | multiply | add | |
| 1, 6 | 1 x 6 = 6 | 1 + 6 = 7 | |
| 2, 3 | 2 x 3 = 6 | 2 + 3 = 5 | Meets requirements |
By relating the algorithm to the visual model, students can see why they are looking for pairs of factors that meet these requirements.
In this second example, I demonstrate zero-sum pairs’ use when building the area necessary to factorise a quadratic expression.
Factorising a quadratic where the coefficient of x2 is not 1
When we change the coefficient of x2, the algorithm described above becomes a little more involved, and students may begin to struggle with factorising. I show how using Algebra Tiles can make this process easier to see in the two videos below.
In Part 5, the last of this series, I will explore perfect squares, completing the square and extending to two-variables.
Resources
There is a Padlet to accompany this blog series with links to research, virtual manipulatives and other resources.